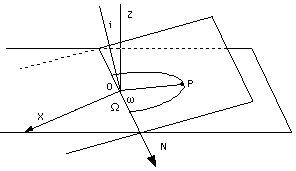
 But what are its actual celestial coordinates (x,y,z)? From the illustration shown here (taken from section #12a), (x,y,z) can be reached by conducting two simple rotations by angles (ω, i, Ω). To connect the two systems, the simple transformation derived above is applied, consecutively--first a rotation around line N by an angle i giving an intermediate system (x",y",z"), after which z' = z" = z. And then another rotation by an angle ω+Ω around the shared z axis, bringing the x' axis, which originally passed the apogee P, into the celestial x axis.
But what are its actual celestial coordinates (x,y,z)? From the illustration shown here (taken from section #12a), (x,y,z) can be reached by conducting two simple rotations by angles (ω, i, Ω). To connect the two systems, the simple transformation derived above is applied, consecutively--first a rotation around line N by an angle i giving an intermediate system (x",y",z"), after which z' = z" = z. And then another rotation by an angle ω+Ω around the shared z axis, bringing the x' axis, which originally passed the apogee P, into the celestial x axis.Special notations exist which simplify the job. Problems involving the orientation of satellites in 3-dimensional space (the "attitude" of those satellites) are handled in a similar way. |